Page 19 - 33-2

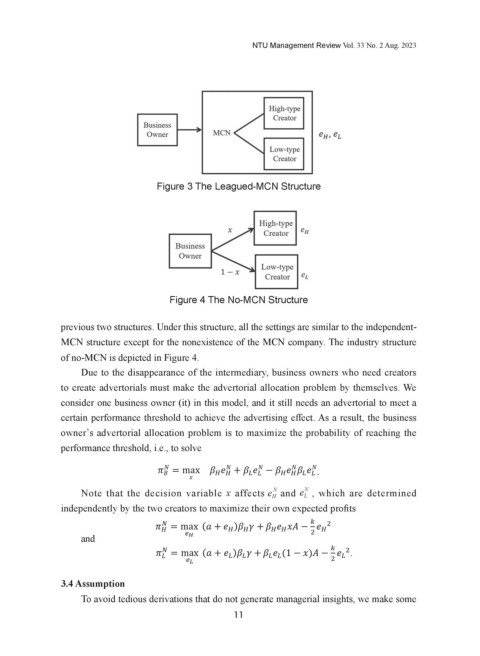
3.3 The No-MCN Structure (N)
Note that while the leagued-MCN structure may serve as a benchmark of the
independent-MCN structure for us to assess the impact of the MCN company, there is
another possibility to do the assessment: completely removing the MCN company.
NTU Management Review Vol. 33 No. 2 Aug. 2023
Therefore, to better figure out the benefit brought by the MCN company, if any, we

consider the third industry structure, the no-MCN structure, which is in between the

previous two structures. Under this structure, all the settings are similar to the

independent-MCN structure except for the nonexistence of the MCN company. The

industry structure of no-MCN is depicted in Figure 4.

Figure 3 The Leagued-MCN Structure

Figure 4. The no-MCN structure
Figure 4 The No-MCN Structure

previous two structures. Under this structure, all the settings are similar to the independent-
Due to the disappearance of the intermediary, business owners who need creators
MCN structure except for the nonexistence of the MCN company. The industry structure
to create advertorials must make the advertorial allocation problem by themselves. We
of no-MCN is depicted in Figure 4.
Due to the disappearance of the intermediary, business owners who need creators
consider one business owner (it) in this model, and it still needs an advertorial to meet
to create advertorials must make the advertorial allocation problem by themselves. We
a certain performance threshold to achieve the advertising effect. As a result, the
consider one business owner (it) in this model, and it still needs an advertorial to meet a
certain performance threshold to achieve the advertising effect. As a result, the business
business owner’s advertorial allocation problem is to maximize the probability of
owner’s advertorial allocation problem is to maximize the probability of reaching the
Note that the decision variable affects
reaching the performance threshold, i.e., to solve and , which are determined

performance threshold, i.e., to solve � �
independently by the two creators to maximize their own expected profits
� � � � .

Note that the decision variable affects and , which are determined
= max
+ −
� �

� �

�
�

N
�
e
Note that the decision variable x affects and , which are determined
N
�
�
e
= max ( )
independently by the two creators to maximize their own expected profits
L
�
�
H � �
�
�
�
� �
independently by the two creators to maximize their own expected profits
15
and �
= max ( ) � �
�
�
�
� �
�
and � � �
= max ( ) (1 ) .
�
�
�
and � � � � � � � � �
3.4 Assumption = max ( ) (1 ) .
�
�
�
3.4 Assumption
�
�
� �
�
�
�
To avoid tedious derivations that do not generate managerial insights, we make some
� �
11
To avoid tedious derivations that do not generate managerial insights, we make
3.4 Assumption
some technical assumptions throughout this study in Assumption 1. These assumptions
To avoid tedious derivations that do not generate managerial insights, we make
are used for all three MCN-creator structures.
some technical assumptions throughout this study in Assumption 1. These assumptions
�
���� ��� � � ��� � �(���)
Assumption 1. Let q = � and q =
�
�
�� � (����)
are used for all three MCN-creator structures. � �(��(���)�) ��
�
.
���� ��� � � ��� � �(���) ��� We assume that β β A < 2k , q ≥0 ,
�
�
���� � � ��� � �(���) �
� � �
�(����)
Assumption 1. Let q = �� and q =
�
�� � (��(���)�)
�
�
�� � (����) � �(��(���)�) ��
� � � � � � � � � � � �
�β A , 4kβ q ≤ 4k β β A , 4k q ≤ (4k
q ≥0 , 4kβ q ≤ 4k β � �(���) � � � � �
�
��� �
�
� �
����
� � ���
� . We assume that β β A < 2k , q ≥0 ,
�� � (��(���)�) � �(����) �� � � �
β β A )(γ xA), and 4k q ≤ (4k β β A )(γ (1 x)A).
� �
�
�
� �
�
�
�
� �
� �
� � � � � � � � � � � �
q ≥0 , 4kβ q ≤ 4k β β A , 4kβ q ≤ 4k β β A , 4k q ≤ (4k
� �
� �
� �
� �
�
�

β β A )(γ xA), and 4k q ≤ (4k β β A )(γ (1 x)A).
� �
�
� �
�
�
�
�
� �
� �
These technical assumptions may be categorized into three groups according to

their major implications. In particular, < 2 , ≥0, and ≥0 are to
�
� �
�
These technical assumptions may be categorized into three groups according to
� � � � � �
make the MCN’s profit function concave, 4 ≤ 4 and 4 ≤
� �
� �
� �
their major implications. In particular, < 2 , ≥0, and ≥0 are to
�
� �
�
4 are to make the probabilities for the creators to meet the target
� �
�
�
� �
� �
�
�
�
�
� �
make the MCN’s profit function concave, 4 ≤ 4 and 4 ≤
� �
� �
� �
and no greater than 1 in equilibrium, and the last two conditions are to make the
� �
4 are to make the probabilities for the creators to meet the target
�
� �
�
� �
� �
revenue sharing ratios and no greater than 1 in equilibrium.
�
�
and no greater than 1 in equilibrium, and the last two conditions are to make the
� �

revenue sharing ratios and no greater than 1 in equilibrium.
�
�
16

16