
臺大管理論叢
第
27
卷第
2S
期
41
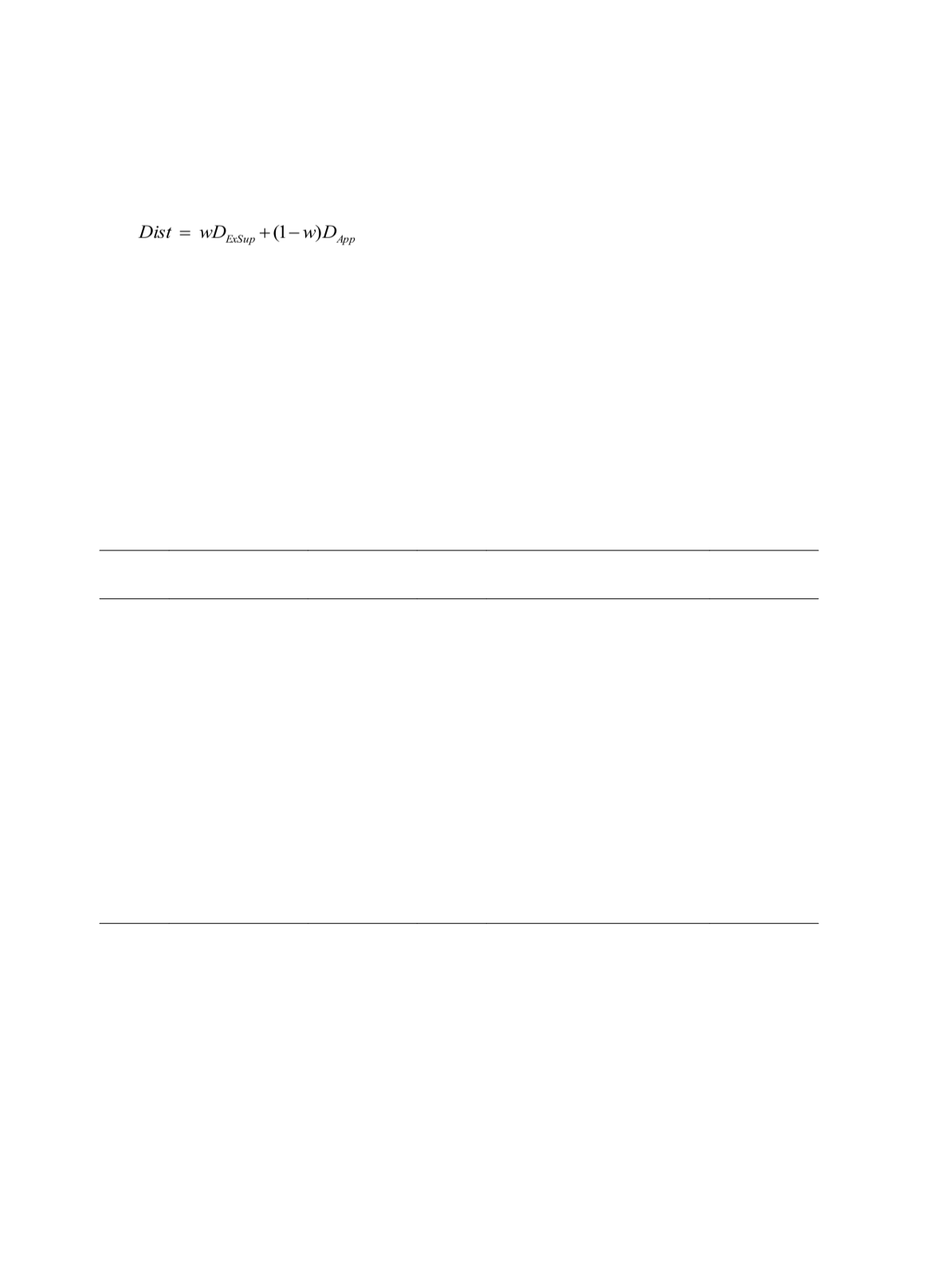
The distance between two FU2Ps is a weighted sum of
D
ExSup
and
D
App
:
(4)
The range of
w
is between 0 and 1. If w is larger than 0.5, the U2 patterns in a cluster
are more likely to have similar expected supports. In contrast, if
w
is smaller than 0.5, the
FU2Ps in a cluster are more likely to have similar appearances.
Table 6 contains a set of FU2Ps, where each FU2P shows its serial number, appearance,
and expected support. In the figure, some FU2Ps have similar appearances but present
significant differences in their expected supports, e.g.,
F
4
and
F
6
. If we set
w
as 0.1 or 0.9,
the distance between
F
4
and
F
6
is 0.0333 or 0.2997. (
D
ExSup
= 0.333 and
D
App
= 0).
Table 6 A Set of FU2Ps
Serial
number
FU2P
Expected
support
Serial
number
FU2P
Expected
support
F
1
[
A
1
:[427, 433]]
1707.000
F
11
[
A
4
:[368, 374]]
3613.998
F
2
[
A
1
:[427, 435]]
1876.000
F
12
[
A
5
:[190, 194]]
3692.028
F
3
[
A
2
:[335, 337]]
2074.049
F
13
[
A
5
:[195, 197]]
1576.016
F
4
[
A
3
:[35, 37]]
1775.109
F
14
[
A
5
:[188, 193]]
3365.006
F
5
[
A
3
:[30, 33]]
1736.733
F
15
[
A
5
:[192, 197]]
2077.285
F
6
[
A
3
:[34, 37]]
2662.734
F
16
[
A
1
:[427, 435],
A
3
:[34, 37]]
1590.300
F
7
[
A
3
:[30, 34]]
2315.562
F
17
[
A
2
:[335, 337],
A
4
:[368, 374]]
1682.998
F
8
[
A
3
:[33, 35]]
3018.757
F
18
[
A
3
:[33, 35],
A
4
:[362, 365],
A
5
:[190, 194]]
1614.752
F
9
[
A
4
:[362, 365]]
1901.214
F
19
[
A
3
:[30, 34],
A
4
:[361, 367],
A
5
:[195, 197]]
1575.002
F
10
[
A
4
:[361, 367]]
2182.797
F
20
[
A
3
:[33, 35],
A
4
:[368, 374],
A
5
:[190, 194]]
3100.002
3.3 The SFC Algorithm
In this section, we present our proposed SFC algorithm. The SFC algorithm adopts a
hierarchical clustering technique to derive clusters of FU2Ps, where the medoid of each
cluster serves as the representative FU2P. Guided by three user-specified parameters, the
SFC algorithm automatically determines the number of clusters. Figure 1 shows the flow
chart of the SFC algorithm.
In the first step, we choose an initial set of representative FU2Ps. In the second step, a
modified
k
-medoids algorithm is applied to the full set of FU2Ps. The procedure of the


















